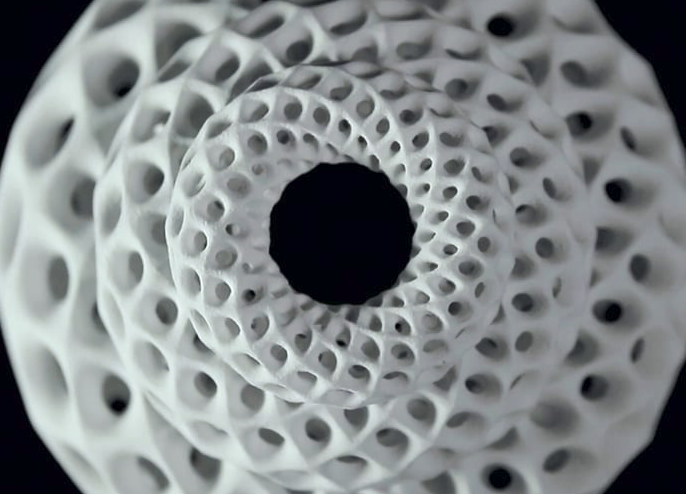
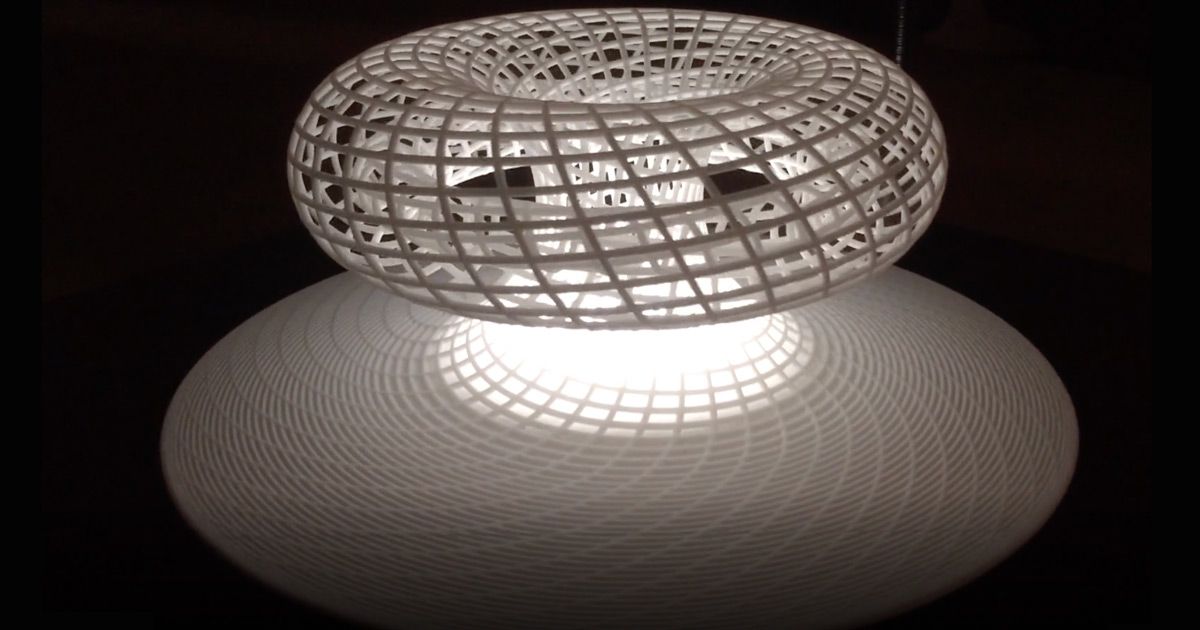
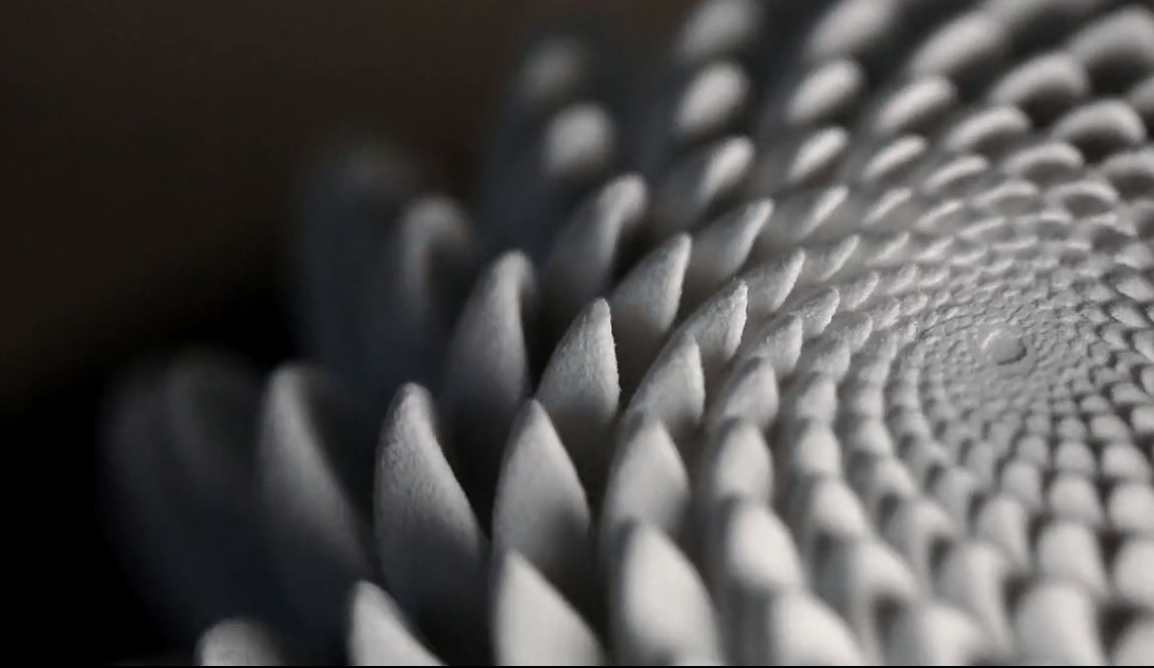
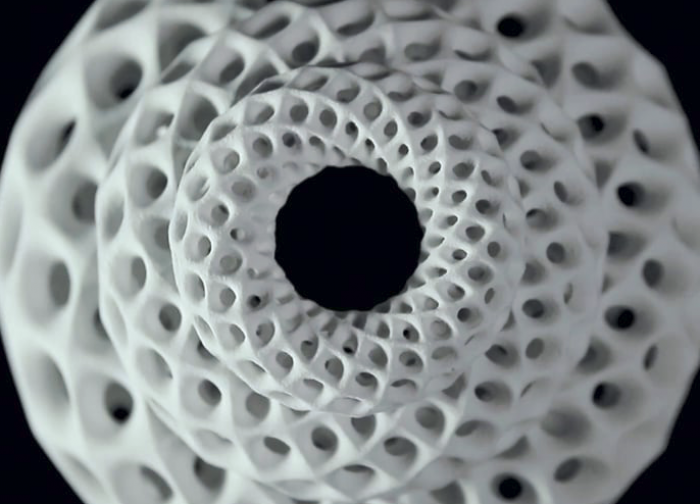
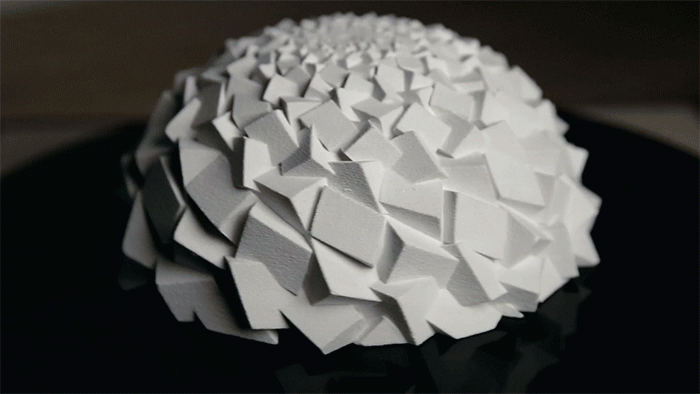
John Edmark, is an inventor, designer and artist who teaches design at Stanford University in Palo Alto, CA. Edmark created a series of 3-D “Fibonacci zoetrope sculptures” that are designed to animate when spun under a strobe light. The placement of the appendages is determined by the same method nature uses in pinecones and sunflowers. The rotation speed is synchronized to the strobe so that one flash occurs every time the sculpture turns 137.5º—the golden angle.
Courtesy of John Edmark
If you count the number of spirals on any of these sculptures you will find that they are always Fibonacci numbers. Each petal on the sculpture is placed at a unique distance from the top-center of the form. If you follow what appears to be a single petal as it works its way out and down the sculpture, what you are actually seeing is all the petals on the sculpture in the order of their respective distances from the top-center.
The illusions generated here are the result of a marriage between art and mathematics. Fibonacci’s Sequence is defined as a recurrent relationship that can be expressed as: F_n = F_{n-1} + F_{n-2} where the first two digits of the sequence can be defined as F_1=1, and F_2=1. What this means is that the sequence starts with two 1’s, and each following digit is determined by adding together the previous two. Therefore, Fibonacci’s Sequence begins: {1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89…} etc.
Courtesy of John Edmark
Edmark explains the concept behind his work as the following : “While art is often a vehicle for fantasy, my work is an invitation to plunge deeper into our own world and discover just how astonishing it can be. In experiencing a surprising behavior, one’s sense of wonder and delight is increased by the recognition that it is occurring within the context of actual physical constraints.
Courtesy of John Edmark
The works can be thought of as instruments that amplify our awareness of the sometimes tenuous relationship between facts and perception. I employ precise mathematics in the design and fabrication of my work. I do this neither out of a desire to exhibit precision per se, nor to exalt the latest technology, but because the questions I’m trying to formulate and answer about spatial relationships can only be addressed with geometrically exacting constructions. Mathematical precision is an essential ally in my goal of achieving clarity.”
While Edmark’s spinning sculptures create the illusion of the objects moving and morphing, the objects themselves are actually rigid forms and do not change in shape. This is a representation of what Edmark is referring to in his statement above when he mentions “the tenuous relationship between facts and perception.”
Courtesy of John Edmark
